Computing Layouts With Deformable Templates
Description
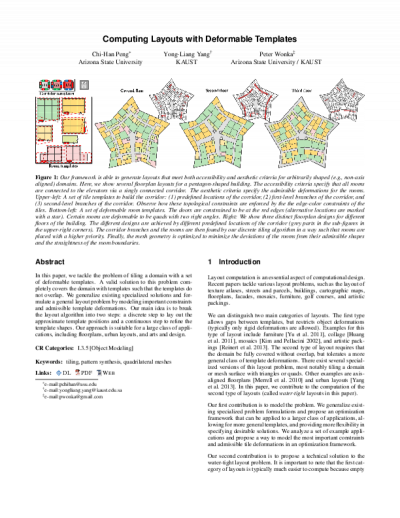
In this paper, we tackle the problem of tiling a domain with a set of deformable templates. A valid solution to this problem completely covers the domain with templates such that the templates do not overlap. We generalize existing specialized solutions and formulate a general layout problem by modeling important constraints and admissible template deformations. Our main idea is to break the layout algorithm into two steps: a discrete step to lay out the approximate template positions and a continuous step to refine the template shapes. Our approach is suitable for a large class of applications, including floorplans, urban layouts, and arts and design.
Date Created
The date the item was original created (prior to any relationship with the ASU Digital Repositories.)
2014-07-01
Agent
- Author (aut): Peng, Chi-Han
- Author (aut): Yang, Yong-Liang
- Author (aut): Wonka, Peter
- Contributor (ctb): Ira A. Fulton Schools of Engineering
