Collective cell migration plays a substantial role in maintaining the cohesion of epithelial cell layers and in wound healing. A number of mathematical models of this process have been developed, all of which reduce to essentially a reaction-diffusion equation with diffusion and proliferation terms that depend on material assumptions about the cell layer. In this paper we extend a one-dimensional mathematical model of cell layer migration of Mi et al. [Biophys. J., 93 (2007), pp. 3745–3752] to incorporate stretch-dependent proliferation, and show that this formulation reduces to a generalized Stefan problem for the density of the layer. We solve numerically the resulting partial differential equation system using an adaptive finite difference method and show that the solutions converge to self-similar or traveling wave solutions. We analyze self-similar solutions for cases with no prolifera- tion, and necessary and sufficient conditions for existence and uniqueness of traveling solutions for a wide range of material assumptions about the cell layer.
Included in this item (4)

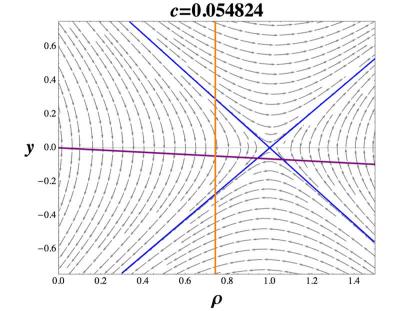
Details
- Traveling Waves in a One-Dimensional Elastic Continuum Model of Cell Layer Migration With Stretch-Dependent Proliferation
- Stepien, Tracy (Author)
- Swigon, David (Author)
- College of Liberal Arts and Sciences (Contributor)
- Digital object identifier: 10.1137/130941407
- Identifier TypeInternational standard serial numberIdentifier Value1536-0040
- Link to published article.
Citation and reuse
Cite this item
This is a suggested citation. Consult the appropriate style guide for specific citation guidelines.
Stepien, Tracy L., & Swigon, David (2014). Traveling Waves in a One-Dimensional Elastic Continuum Model of Cell Layer Migration with Stretch-Dependent Proliferation. SIAM JOURNAL ON APPLIED DYNAMICAL SYSTEMS, 13(4), 1489-1516. http://dx.doi.org/10.1137/130941407

